Jacob Cruger, David Shindell, & Kurtis So

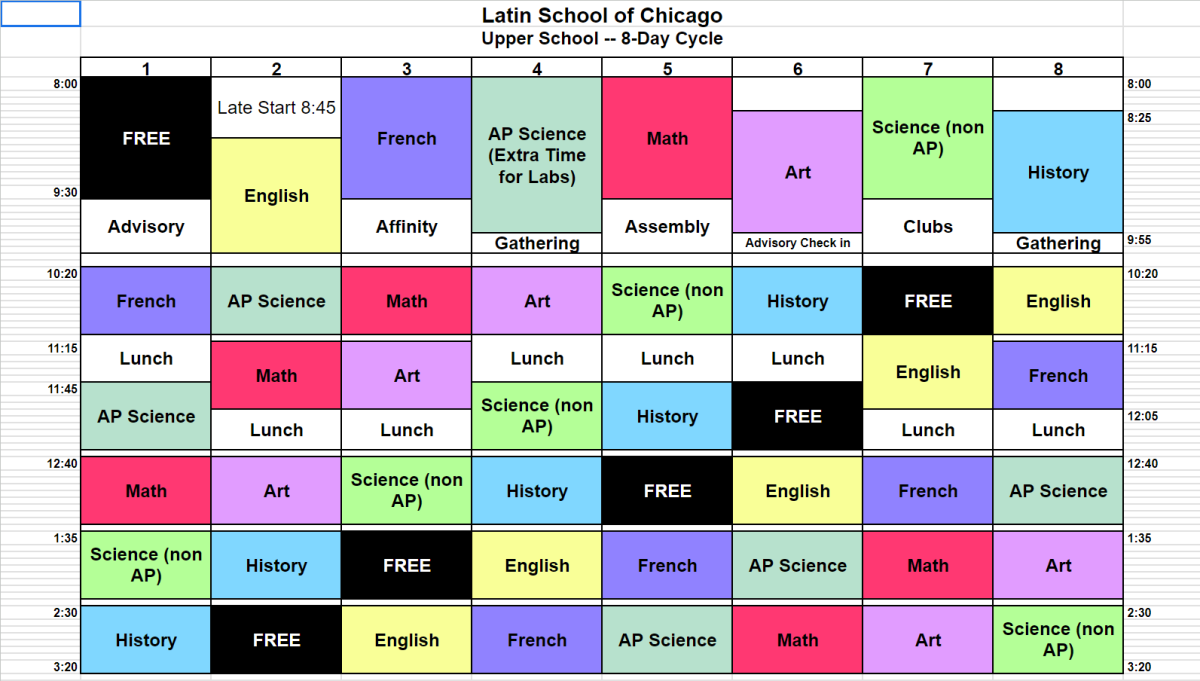
Last year in October the Independent School Health Survey was conducted in the Upper School, asking every student a series of questions via an online platform. It requested that the students be as honest as possible in their responses. One of the questions asked students whether or not they had previously cheated on a test or a quiz – 80% of students who took the survey responded that they had never cheated on a quiz nor on a test. Several students in Ms. Dhaliwal’s AP class sought to test the validity of this result

We selected our own sample by randomly choosing one advisory from each grade to survey. Our survey asked three questions: “How many tests have you cheated on in high school?”, “How many quizzes have you cheated on in high school?”, and “Have you ever been enrolled in another high school?” Originally; the first two questions were meant to be quantitative, as we asked our survey takers to write down a number; while the third was a simple yes or no question. However, because of complications with our survey results, we were forced to convert our responses into binary data. Instead of using a numerical value, we organized our questions into “Have you ever cheated on a test before in high school?” and “Have you ever cheated on a quiz in high school?” and counted any value other than zero as a “Yes.” Despite having to make that change, we were still able to test the claim in question. We intended to use a significance test for population proportions to determine whether or not the data in our sample provided evidence that corroborated or refuted the results of the school’s health survey.

The fraction of students in our sample who admitted to cheating was equal to .13 (13%), significantly lower than the expected value predicted by the survey, which was .2 (20%). In order to determine whether or not this sample’s results refuted the claims of the health survey, we had to calculate the probability of getting that result assuming the Health Survey findings were true. If that probability came out to less than 5%, a generally accepted threshold for statistical significance, we would know that we had evidence suggesting that the Health Survey finding was indeed false. Following the accepted protocol for a significance test of this sort, we arrived at a probability of .1762. This value suggested that the result of our sample was entirely plausible, providing no evidence refuting the claims made by the health survey. This outcome was not surprising; because the school-wide survey collected data from the entire population (thus removing the error inherently associated with smaller random samples), it was reasonable to assume that its results would closely match the actual value for the proportion of Latin students who had cheated, despite the possibility of students being dishonest in their answers.